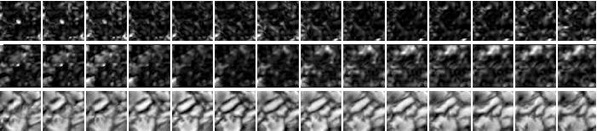
Acoustic Oscillations

The image shows short sequence of the granular evolution and accompanying acoustic power registered on two different heights. The bottom row shows granulation, middle one shows the acoustic power registered at the height of 200 km above the white light level, while the top one shows the acoustic power registered at the height of 600 km.
Most of the prominent power follows the granular evolution.
The acoustic oscillations are stochastically excited by turbulent convection in sub-photospheric layer. (Goldreich & Kumar 1988). It is shown that individual sunquakes are source of the power that is being delivered into the resonant modes of the Sun. (Goode et al. 1998)
Although there is a general consensus that the source of acoustic oscillations is convective motion, some authors argue that one cannot decide whether their origin is convective or magnetic. The observational and theoretical results do not reveal a clear picture of the source of solar oscillations (Moretti et al. 2001).
Observations (Goode et al. 1992; Rimmele et al. 1995; Espagnet et al. 1996) show that the acoustic events occur preferentially in the dark intergranular lanes, i.e. corresponding to down flows of plasma. Goode concluded that the source of the oscillations is located less than 200 km below the photosphere and Rimmele connects the excitation of the solar oscillations more closely with the rapid cooling occurring in the upper convection layer, rather than the overshooting of turbulent convection itself. Espagnet concluded that the excitation of solar oscillations is associated with a rapid cooling occurring in the upper convection layer, and that the most energetic oscillations are associated with down-flows in dark areas that are well separated from each other in time and space (Espagnet et al. 1992). There is observational evidence that acoustic oscillations tend to be converted into magneto-acoustic oscillations at locations where a magnetic field is expected, e.g., at granular boundaries or in bright points (Espagnet et al.1992).